正射影

原点を通る直線 \(l\) を考え、直線 \(l\) の方向ベクトルを \( \mathbf{a} \) とする。このとき、座標上任意の点 \( \mathbf{x} \) から、直線 \( l \) 上に下ろした垂線の足を \( \mathbf{x}' \) とすると、\( \mathbf{x}' \) を \( \mathbf{x} \) の \( \mathbf{a} \) への正射影という。正射影を転換 T を用いて \(\mathbf{x}' = T(\mathbf{x})\) と書くことがある。

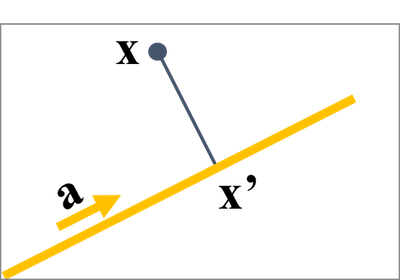
\( \mathbf{x}' \) は直線 \(l\) 上にあるから、\(\mathbf{x}' = t\mathbf{a}\) となる実数 \(t\) が存在する。実数 \(t\) は、\(\mathbf{x} \) と \( \mathbf{x}' - \mathbf{x} \) が垂直であることを利用して、求めることができる。

\[ \begin{eqnarray} (\mathbf{x}, \mathbf{x}' - \mathbf{x}) &\Longleftrightarrow& (\mathbf{a}, t\mathbf{a} - \mathbf{x}) = 0 \\ &\Longleftrightarrow& (\mathbf{a}, t\mathbf{a}) - (\mathbf{a}, \mathbf{x}) = 0 \\ &\Longleftrightarrow& t(\mathbf{a}, \mathbf{a}) - (\mathbf{a}, \mathbf{x}) = 0 \\ &\Longleftrightarrow& t = \frac{(\mathbf{a}, \mathbf{x})}{(\mathbf{a}, \mathbf{a})} \end{eqnarray} \]　

正射影の性質

\(\mathbf{a}\) と \(\mathbf{b}\) を \(\mathbf{0}\) でない平面ベクトルとし、\(\mathbf{a}\perp \mathbf{b}\) とする。また、変換 \(T(\mathbf{x})\) を \(\mathbf{x}\) の \(\mathbf{a}\) への正射影とし、変換 \(S(\mathbf{x})\) を \(\mathbf{x}\) の \(\mathbf{b}\) への正射影とする。このとき、以下のことが成り立つ。

任意の平面ベクトル \(\mathbf{x}\) に対して、\( T(T(\mathbf{x}))=T(\mathbf{x}) \) である。
任意の平面ベクトル \(\mathbf{x}\) に対して、\( S(T(\mathbf{x}))=\mathbf{0} \) である。
任意の平面ベクトル \(\mathbf{x}\) に対して、\( T(\mathbf{x}) + S(\mathbf{x}) = \mathbf{x} \) である。